Det=0 Unique Solution

2 x1 x4 carbon 2x 1 - x 4 0 ---- 1 6x1 2x3 Hydrogen 6x1 - 2x3 0 ---- 2 2x2 1x3 2x4 Oxygen 2x2 - x 3 - 2x 4 0 ---- 3 rank of A is 3 rank of A B 3 4. In mathematics the determinant is a scalar value that is a function of the entries of a square matrixIt allows characterizing some properties of the matrix and the linear map represented by the matrix.

Determinants Online Presentation

0 2 1 l cy dx l ay bx As with Case I the slope of l 1 is a b m 1 the slope of l 2 is c d m 2.

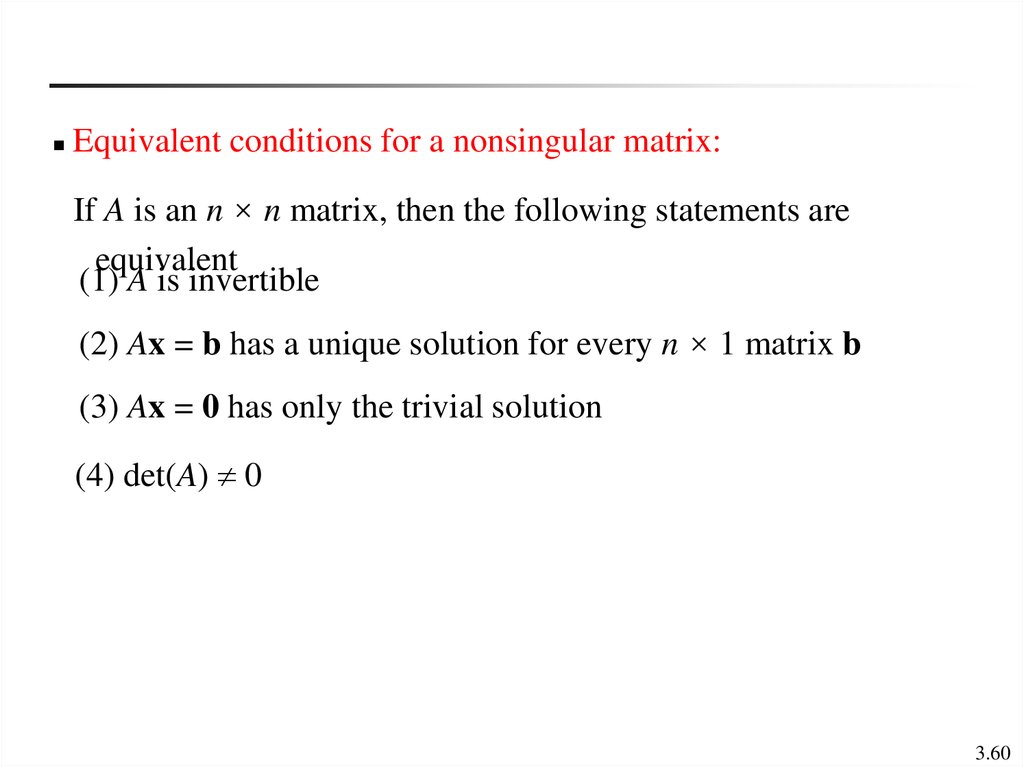
Det=0 unique solution. U is said to be linearly independent provided the equation la mb. Det 0 c d a b the system has a unique solution. Indeed it is possible to deﬁne detA for a square matrix A of arbitrary dimension.

If A is row equivalent to B then the systems Ax 0 and have the same solution. If det 0 c d a b the system may have no solution or infinitely many solutions. 22 Linear independence and basis vectors A set of vectors ab.

Which impossible 0 cannot equal -3. Let Ddenote the value of the determinant. Compatible if it has at least one solution.

Ann 1 C A 0 2 the system has a unique solution given by Kramers rule. Use Cramers rule to ﬁnd the solution x to the system of equations whose matrix equation is given by 3 0 1 1 1 0 1 0 1 x1 x2 x3 2 0 1. The number of variables in the coefficient part of is equal to the number of nonzero rows equal to the in the.

Show that the zero vector 0 is unique and that for each a there is only one inverse a. If A and Bare n x n matrices and A is invertible then ABA B 3. Su 0 has no solution except l m.

There are two cases actually. A system that has no solution is called incompatible. Then D det 0 12 6 0 11 5 1 10 2 2 1 A Given.

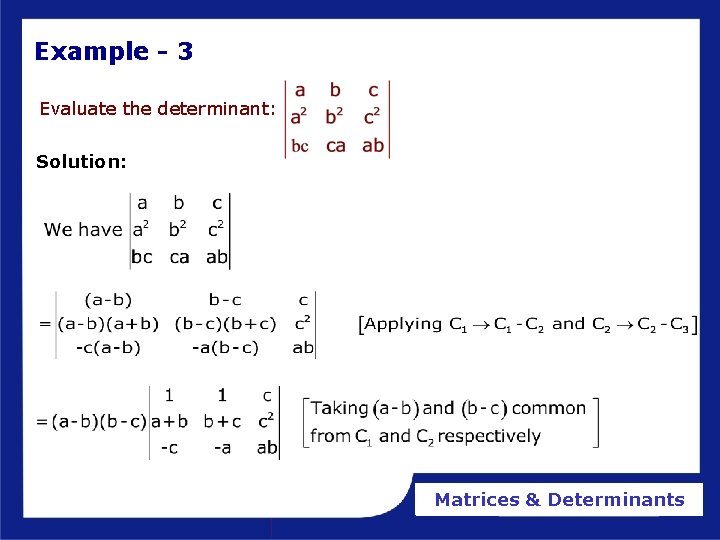
In particular the determinant is nonzero if and only if the matrix is invertible and the linear map represented by the matrix is an isomorphismThe determinant of a product of matrices is. When an order on the unknowns has been fixed for example the alphabetical order the solution may be described as a vector of values like 3 2 6 displaystyle 3-26 for the previous example. 6det 0 0 1 2 1 1 1 0 3 2 1 A combo131 combo212.

The rule says that the solution to this system is given by x1. A unique solution X. If det Aメ0 then Ax-b has a unique solution for every b in Rn 2.

So we get a linear homogenous equation. Unique solution means det 0 for both no solutions and infinite solutions det 0 to clarify let us imagine two random lines. Check the conditions that GUARANTEE that det A 0.

Or det A 0 in which case dim N A 0 rank A n and the system has an inﬁnite number of solutions. 1 Issue 6 August - 2012 ISSN. Ax by e ---- gradient -ab cx dy f ---- gradient -cd.

If the determinant of system 1 is nonzero det 0 B a11 a12. In this case the unique solution is described by a sequence of equations whose left-hand sides are the names of the unknowns and right-hand sides are the corresponding values for example. For the one value of alpha where detA 0 I would use row reduction on the system to find the values of beta that produce an inconsistent no solutions or consistent infinite solutions system.

6det 0 1 1 1 0 swap121 2. The state transition matrix is the unique solution to 0 0 tt A t t t t t t I 00 n 2 International Journal of Engineering Research Technology IJERT Vol. Thus det A 0 implies that the system has a unique solution On the other hand if from MATH 3321 at University of Houston.

In other words check the box if. Since the coeﬃcient matrix A 3 0 1 1 1 0 1 0 1 is square and invertible weve already calculated that detA 2 we can apply Cramers rule to the problem. X1 1 x2 2 xk k xn n.

DimNA 0 and the system has a unique solution. For our purposes we do not so much wish to give a rigorous deﬁnition of such a determinant as. Import numpy as py from scipylinalg import solve A 1 1 1 0 1 -3 2 1 5 b 2 1 0 x solve Ab x.

Therefore this system of linear equations has no solution. The number of variables in the coefficient part of is more than the number of nonzero rows in the last augmented column of. Lets use python and see what answer we get.

Then the system is consistent and it has infinitely many solution. Det 0 12 6 0 1 1 1 terminant unchanged2 4 2 1 A combo12-1 combo13-1. Combination leaves the de- 6det 0 2 1 0 on row 1 factors out a1 Multiply rule1 1 2 4 2 1 A m 16 6.

Ii Infinitely many solutions. Hoping this can be a good starting point for you. Infinitely many solutions X.

If the b is in the column space of A and since det A0 then it will have infinitely many solutions. S 0. If the det A 0 then the system of linear equations represented by AX B could possibly have.

In such a way that if detA 6 0 then the system has a unique solution situation 1 but if detA 0 then one of the other situations 24 is in eﬀect. If the vector b is not in the column space of the matrix A it will have no solutions. All values where detA is not 0 are unique solutions.

Pin On Mathematics

Chapter 1 Matrices Determinants Session Objectives Meaning Of

How To Multiply Two Different Numbers In Fastest And Quickest Ways Simplifying Mathematics In Simple Way Int Multiplication Tricks Math Tricks Maths Puzzles